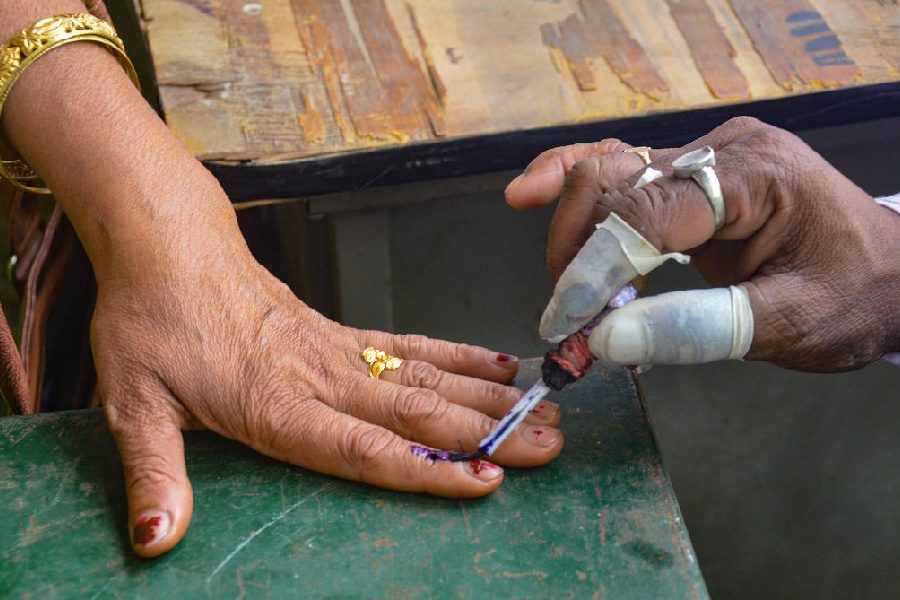
Friday, 19 April 2024
Friday, 19 April 2024
 Friday, 19 April 2024
Friday, 19 April 2024













Mum-to-be Deepika picks up a new hobby
‘I Am: Celine Dion’, the much anticipated documentary, is set to premiere on Prime Video
Drop by at the Italian Food Festival at Ecohub this weekend
Feashts recreates an iconic dish from an iconic film
Say, ‘Hello, Lover!’ with Sameer Madan's new summer collection
The 1990s was a watershed era. A new entity called non-government organisation emerged at the grassroots level which redefined the nature of non-party political formations
HILAL AHMED

Last rites and rituals are the last to change. My conversations with ritual specialists reveal that some changes are taking place. We are exploring greener cremation systems
MINAKSHI DEWAN
When the trajectories of languages get snapped, the accumulated wisdom in those languages, too, gets submerged and continues to survive in severely truncated, irreparable and insensible forms
G.N. DEVY
The manipulation of brain activity has also been around for some time now and has been ramped up with Artificial Intelligence-based ‘interventions’
PRAMOD K. NAYAR
Deep, entrenched divisions over the way forward are holding back prospects of peace. With 'compromise' a bad word, the Russia-Ukraine conflict risks turning into a forever war
THE EDITORIAL BOARD































Adah Sharma features in Sunflower Season 2, which is streaming on ZEE5